Wherein I list some (mostly) recent happenings, ramble a bit, and provide links, in an order roughly determined by importance and relevance to particle physics. Views are my own. Content very definitely skewed by my own leanings and by papers getting coverage, and it may not even be correct. It is a blog after all...
- CMS has submitted their paper on the search for LFV Higgs decays. This analysis supersedes the PAS from July (which has 20 citations). They see an excess of 2.4σ, which I wouldn't make much of, but it is worth keeping track of these "hints" because they motivate papers you see popping up on hep-ph. The following is a quick summary...
They search in the $\mu\tau$ channel, which is experimentally the best channel in which you might hope to see something after taking into account bounds from LFV transitions. The best fit branching ratio for the excess corresponds to $Br(H\to \mu\tau)=0.84\%$, an order of magnitude below bounds from $\tau\to\mu\gamma$. The dominant backgrounds for the search are $Z\to\tau\tau$, $W+jets$, QCD multijets, and $t\bar{t}$. The latter three are certainlysome of the nastiest backgrounds around at the LHC. $W+jets$ in particular is responsible for a number of historical anomalies (see Tomasso's blog or his talk). Happily they can be estimated with the help of data in background-enriched regions, which is nice, but shape uncertainties must then be carefully considered as systematics. Anyway, let us move on to the results...
The left and right columns correspond to the leptonic and hadronic $\tau$ decay channels respectively, and each row corresponds to an increasing number of jets (the last row is enhanced with VBF events). The variable they plot against is the collinear mass $M_{col}$, an estimator for the Higgs mass (in the signal events) constructed by assuming the neutrino (coming from the $\tau$ decay) momentum is equal to the projection of the missing momentum vector onto the direction of the $\tau$ decay products. The distributions driving the excess are $\mu\tau_e\;0$-jet, $\mu\tau_e\;1$-jet, and $\mu\tau_h\;2$-jet. That can be seen by eye above and in the branching fits themselves:
One would assume (or hope?) that the ATLAS analysis is underway, and certainly it will be interesting to find out what they see.
- ATLAS has bounded the Higgs width at < 22.7 MeV at 95% CL (SM is 4.1 MeV) using the off-shell $gg\to VV$ Higgs boson signal, with assumptions (see below)! This supersedes their Conf Note from July and adds the WW channel to the analysis. The idea of doing this measurement (as far as I know) is based on the paper from Caola/Melnikov (1307). CMS managed to do the analysis and present it as a prepublication within 6 months of that paper (a phenomenal effort), rushed out for Moriond [pdf], and later published (in September) a bound of < 22 MeV at 95% CL [it should be noted that in the present ATLAS analysis the CLs method has been used which weakens their upper bound in the presence of the observed downward fluctuation of the low-statistics background; CMS used the regular $-2\ln L<4$ method and if ATLAS had done the same, judging by their Figure 12, their bound would rather be < 16.4 MeV]. The CMS result was presented as having "mild model-dependence," but not long after the bound was shown to be invalid for general new physics scenarios without some specific assumptions (see e.g. Englert/Spannowsky 1405). ATLAS appear to have specified clearly the assumptions going into their analysis...
The ratio of the off-shell to on-shell g-g fusion signal strength is $$\frac{\mu_{\text{off-shell}}(\hat{s})}{\mu_{\text{on-shell}}}=\frac{\kappa^2_{g,\text{off-shell}}(\hat{s})\kappa^2_{V,\text{off-shell}}(\hat{s})}{\kappa^2_{g,\text{on-shell}}\kappa^2_{V,\text{on-shell}}}\frac{\Gamma_H}{\Gamma_H^{SM}},$$where the $\kappa$ are coupling scale factors of the Higgs to $gg$ and $VV$. The approximation CMS made was that the ratio of $\kappa$'s was equal to unity. ATLAS sets their limit under the assumption $$\kappa_{g,\text{on-shell}}^2\kappa_{V,\text{on-shell}}^2\le \kappa_{g,\text{off-shell}}^2\kappa_{V,\text{off-shell}}^2 .$$They also assume that none of any new physics which might alter the Higgs width changes substantially the background (of particular interest in this case since the off-shell signal and continuum background destructively interfere). The higher-order QCD corrections for the continuum background are not available, so in presenting their results they allow this K-factor to vary by a factor of 2 around that calculated for the SM Higgs.
I find this measurement interesting as an independent way to probe the Higgs width. In principle it is possible to increase the Higgs width to > 22 MeV and have it consistent with all measurements, but it involves scaling up SM couplings while adding a new decay mode to keep the production×decay rate fixed. The global Higgs fit means that the upper bound on the Higgs branching to unobserved (not necessarily invisible) decays is < 21% for an otherwise SM Higgs.
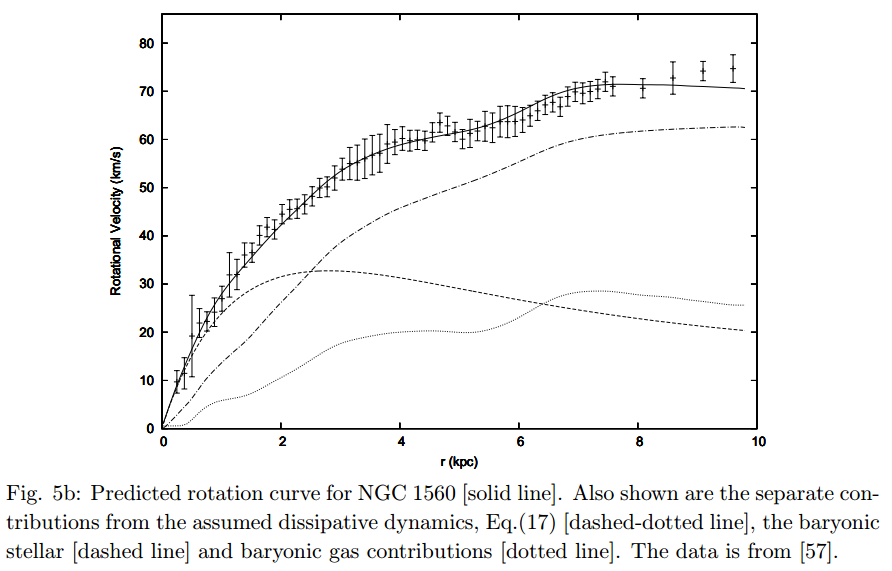
- An arXiv preprint has shown that a one-parameter generic dissipative dark matter model with supernovae heat source can explain the 'wiggles' in rotation curve data. Assuming that DM cooling is balanced by this heating mechanism and the DM density is in a stable state, it is shown that the density of DM is related to the supernovae formation rate in the disk. If the SN rate is related to the gas density via a Kennicutt-Schmidt law, then the DM density is connected to the baryonic gas density in the disk: $$\rho(r,\theta)=\tilde\lambda \int d\tilde\phi \int d\tilde r \tilde r \frac{[\Sigma_{gas}(\tilde r)]^N}{4\pi[r^2+\tilde r^2-2 r \tilde r \sin\theta\cos\tilde\phi]},$$where $N\approx 2$, and $\tilde\lambda$ is an appropriately averaged quantity which depends on the cross section, supernovae dark photon energy spectrum etc.
You can now go out and apply this equation to gas density measurements and predict the rotation curve up to the constant $\tilde\lambda$. This was done for the spiral galaxy NGC1560 (below):
There are more examples in the paper, fitting to dwarf galaxies from the
LITTLE THINGS survey released last month. Reasonably good fits are obtained, especially considering it is only a one-parameter model; the best fit values of $\tilde\lambda$ vary only within a factor of two.
- The PICO-2L C3F8 Bubble Chamber in SNOLAB have reported the most sensitive direct detection constraints on WIMP-proton spin-dependent scattering to date. Below is a plot which compares various limits.
The limits which appear to "beat" PICO-2L come along with some assumptions: IceCube, ANTARES, and SuperK are neutrino telescopes looking for annihilating dark matter in the sun; CMS/ATLAS search for mono-X signatures and assume an effective field theory (valid if the mediator mass is $\gg\sqrt{\hat{s}}$). In the conclusion they claim "These limits represent... the first time supersymmetric parameter space has been probed by direct detection in the SD-proton channel." Can't help but doubt this...
- The Neutrino Telescopes conference (NeuTel XVI) is well under way and is keeping a blog here which contains summaries of talks and posters. I really like this method for gathering in one place succinctly and accessibly the important information; of course one can still access the full talks to find out more.
Here is one interesting post from Francesco Iachello. He claims that quoted neutrinoless double beta decay bounds are too strong by a factor 2.5--6 due to the overestimate of a nuclear matrix element factor. This would mean that the inverted hierarchy region still allowed by Planck cannot be probed by $0\nu2\beta$ experiments in near future.
- There is an interview with Jamie Bock of the BICEP2 experiment at Sean Carroll's blog. Here is an excerpt on the decision to release results when they did: The question really is, should we have waited until better data were available on galactic dust? Personally, I think we did the right thing. The field needed to be able to react to our data and test the results independently, as we did in our collaboration with Planck. This process hasn’t ended; it will continue with new data. Also, the searches for inflationary gravitational waves are influenced by these findings, and it is clear that all of the experiments in the field need to focus more resources on measuring the galaxy.
- Twenty years ago on Tuesday was the discovery of the top quark at Tevatron. The papers of CDF and D0 were released together and can be read for free at PRL Milestones. Top at Twenty at Fermilab is celebrating this milestone from 9-10 April, in the traditional way of particle physicists: talks! Reviews of fundamental measurements of the top quark, measurements of top quark production and decay, theoretical talks on how the top quark fits into the Standard Model and its potential extensions, etc...
- I don't know how significant this tweet from today is, but perhaps something to be aware of... [Edit: never mind, must have been minor (see second tweet)]
- A paper published yesterday in Science has measured the D/H enrichment of atmospheric water on Mars. They found a D/H value enriched by a factor of about 7 relative to Earth’s ocean, which indicates that Mars has lost most of its water to space -- about six times the amount presently locked up in the Martian ice caps. This implies that Mars once had an ocean covering 20% of the planet's surface, up to one mile deep, and was wet for >1.5 billion years. Plenty of time for life! There's a 4 minute video about it from NASA Goddard, or an article at the guardian if you prefer.
- Another paper in Science reports the observation of a gravitationally lensed supernova forming an Einstein cross. Because of the different travel times for light rays taking different paths around the lensing cluster, it is suspected that the supernova explosion would have appeared before (1964 and 1995) in another part of the sky, and will appear again elsewhere some time before 2020. There's an NY Times article here with a video.
- Here is Rolf Heuer on the European Commission's decision to divert €2.7 billion (4%) of the EU’s science funding programme, Horizon 2020, to alternative investments.
- Not sure what to think of the following tweet from Murdoch, but with The Australian's recent flip-flopping on Abbott who knows...
- If you aren't already following Sabine Hossenfelder blogging at BackReaction, you should. Two more interesting pieces from her this week: 1) Are pop star scientists bad for science? 2) Can we prove the quantization of gravity with the Casimir effect? Probably not.
- Very many articles knocking around this week about Homer Simpson predicting the Higgs boson mass, apparently relating to Simon Singh's new(ish) book on Mathematics and The Simpsons. Actually, you can now read that chapter for free here.
If you actually calculate it you find it to be $\approx 775$ GeV. Not so miraculous. Change the $\pi$ to a $\frac12$ and he does a lot better, but since we know that the Higgs mass is just a conspiracy of gravity, QCD, and the weak force, maybe he should have guessed $$ \approx \pi\left(\frac{m_p}{2m_W}\right)^8\sqrt{\frac{hc}{G}}, $$ at tree level of course.
- Lastly, images from space...
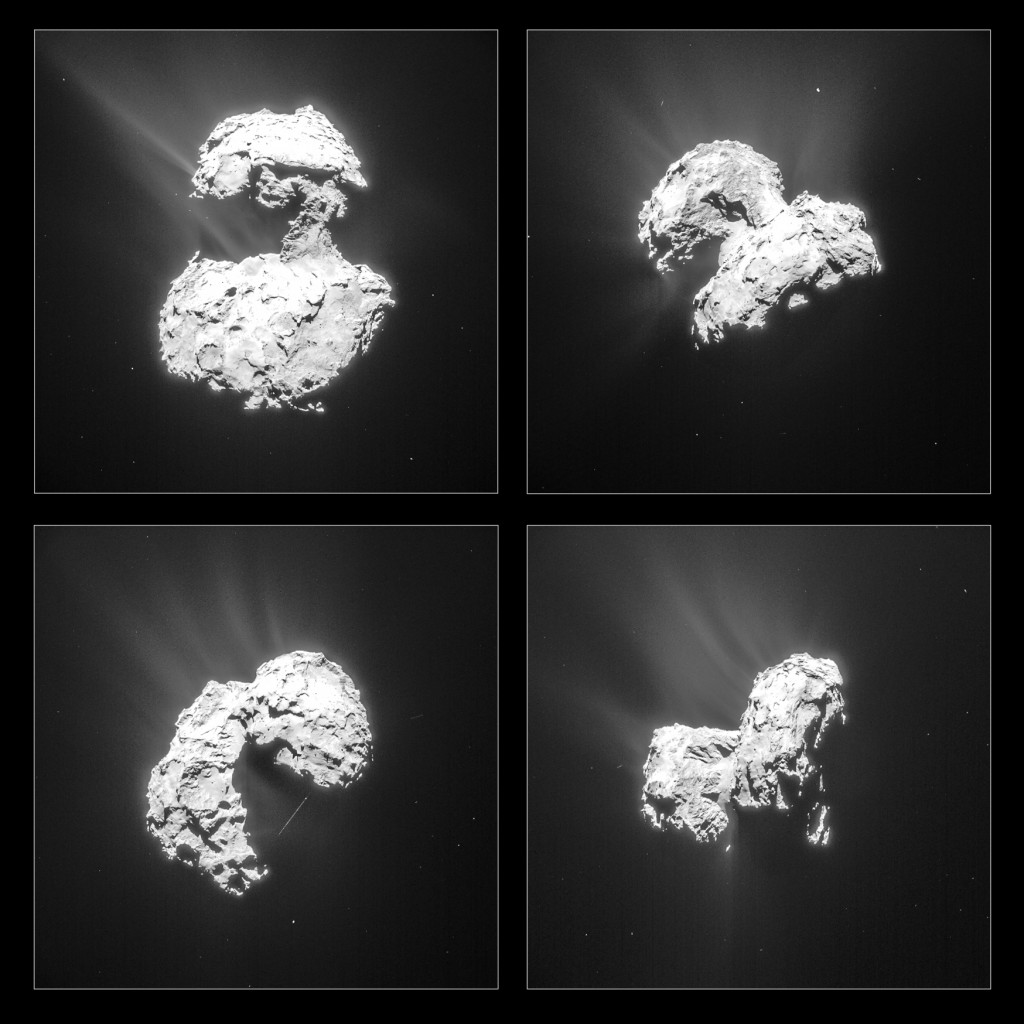
- Some shots from Rosetta: all-round activity and and image of its own shadow captured during the recent comet flyby!
- Dawn is approaching Ceres. It should go into orbit some time today! See the video section above to look at the manoeuvre that will get it there.
One would assume (or hope?) that the ATLAS analysis is underway, and certainly it will be interesting to find out what they see.
The limits which appear to "beat" PICO-2L come along with some assumptions: IceCube, ANTARES, and SuperK are neutrino telescopes looking for annihilating dark matter in the sun; CMS/ATLAS search for mono-X signatures and assume an effective field theory (valid if the mediator mass is $\gg\sqrt{\hat{s}}$). In the conclusion they claim "These limits represent... the first time supersymmetric parameter space has been probed by direct detection in the SD-proton channel." Can't help but doubt this...
No comments:
Post a Comment